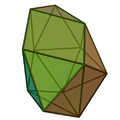
加长型球状屋顶
外观
 | |||
| 类别 | 约翰逊多面体 J87 - J88 - J89 | ||
|---|---|---|---|
| 识别 | |||
| 名称 | 加长型球状屋顶 sphenomegacorona | ||
| 别名 | 長球形屋根(日语) | ||
| 参考索引 | J88 | ||
| 鲍尔斯缩写 | wamco | ||
| 性质 | |||
| 面 | 18 | ||
| 边 | 28 | ||
| 顶点 | 12 | ||
| 欧拉特征数 | F=18, E=28, V=12 (χ=2) | ||
| 组成与布局 | |||
| 面的种类 | 16个三角形 2个正方形 | ||
| 顶点图 | 2个(34) 2个(32.42) 2×2个(35) 4个(34.4) | ||
| 对称性 | |||
| 对称群 | C2v群 | ||
| 特性 | |||
| 凸 | |||
| 图像 | |||
| |||
加长型球状屋顶(日语:長球形屋根、英语:Sphenomegacorona)是一种由16个三角形和2个正方形组成的十八面体[1],为约翰逊多面体的其中一个,索引为J88[2]。它无法由帕雷托立体(正多面体)和阿基米得立体(半正多面体)经过切割、增补而得来,是约翰逊多面体中的基本立体之一。约翰逊多面体是凸多面体,面皆由正多边形组成但不属于均匀多面体,共有92种。这些立体最早在1966年由诺曼·约翰逊(Norman Johnson)命名并给予描述[3]。
性质
[编辑]加长型球状屋顶共由18个面、28条边和12个顶点所组成[4][5][6][7]。在其18个面中,有16个正三角形和2个正方形[5]。在其12个顶点中,有2个顶点是4个正三角形的公共顶点[7],在顶点图中可以用[34]来表示[8]、还有4个顶点是5个正三角形的公共顶点[7],在顶点图中可以用[35]来表示[8]、还有4个顶点是4个正三角形和1个正方形的公共顶点[7],在顶点图中可以用[34,4]来表示[8]、剩下的2个顶点是2个正三角形和2个正方形的公共顶点[7],在顶点图中可以用[32,42]来表示[8]。
体积与表面积
[编辑]而其体积为:
其中的常数由![]() A334114给出[11],其为下列多项式的其中一个实根,约为1.948108228859[11]:
A334114给出[11],其为下列多项式的其中一个实根,约为1.948108228859[11]:
顶点坐标
[编辑]边长为2的加长型球状屋顶的顶点坐标为:
其中,、和为:
其中, ≈ 0.59463是下列多项式的做小实根:
这些坐标也可以由下列顶点的轨道的并集在沿xz平面和yz平面镜射所产生的空间对称群之群作用下给出:[12]
相关多面体
[编辑]-
加长型球状屋顶
(正方形附近的4个位置上各加上1个正三角形的球状屋顶) -
五角锥球状屋顶
(合并两个移除了两个正三角形的球状屋顶)
参见
[编辑]参考文献
[编辑]- ^ Santiago Alvarez. Polyhedra in (Inorganic) Chemistry (PDF). Electronic Supplementary Information for Dalton Transactions. 2005 [2022-09-25]. (原始内容存档 (PDF)于2022-01-21).
- ^ Weisstein, Eric W. (编). Sphenomegacorona. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).
- ^ Johnson, Norman W., Convex polyhedra with regular faces, Canadian Journal of Mathematics, 1966, 18: 169–200, MR 0185507, Zbl 0132.14603, doi:10.4153/cjm-1966-021-8.
- ^ V.Bulatov. sphenomegacorona. [2022-09-11]. (原始内容存档于2022-12-08).
- ^ 5.0 5.1 David I. McCooey. Johnson Solids: Sphenomegacorona. [2022-09-07]. (原始内容存档于2022-09-11).
- ^ The Sphenomegacorona. qfbox.info. [2022-09-11]. (原始内容存档于2023-01-03).
- ^ 7.0 7.1 7.2 7.3 7.4 Sphenomegacorona. polyhedra.tessera.li. [2022-09-11]. (原始内容存档于2022-12-05).
- ^ 8.0 8.1 8.2 8.3 Richard Klitzing. sphenomegacorona, wamco. bendwavy.org. [2022-09-11]. (原始内容存档于2022-12-08).
- ^ Wolfram, Stephen. "Sphenomegacorona". from Wolfram Alpha: Computational Knowledge Engine, Wolfram Research (英语).
- ^ Wolfram Research, Inc. Wolfram|Alpha Knowledgebase. Champaign, IL. 2020.
PolyhedronData[{"Johnson", 88}, "SurfaceArea"] - ^ 11.0 11.1 Sloane, N.J.A. (编). Sequence A334114. The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- ^ Timofeenko, A. V. The non-Platonic and non-Archimedean noncomposite polyhedra. Journal of Mathematical Science. 2009, 162 (5): 720. S2CID 120114341. doi:10.1007/s10958-009-9655-0.